Volume to Density Calculator

Volume to Density Calculator - Calculate mass and density from volume

Weight/Mass (kg):

Weight/Mass (g):

Weight/Mass (lb):

Weight/Mass (oz):

Density (kg/m³):

Density (g/cm³):

Density (oz/cu in):

Density (lb/cu in):

Density (lb/cu ft):

Density (lb/cu yd):

What is Volume to Density

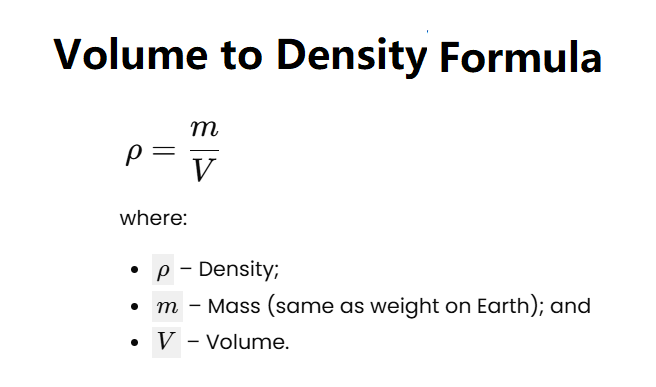
Volume to density refers to the process of calculating the density of a substance based on its volume and either its mass or a known density. Density is defined as \(\rho = \frac{m}{V}\), where \(m\) is mass and \(V\) is volume. If the mass is known, the density can be directly calculated. Alternatively, if a density is provided, the mass can be derived as \(m = \rho \cdot V\). This calculator allows you to input a volume and optionally a density to compute the corresponding mass and density.

For example, with a volume of 15 m³ and a mass of 22 kg (as shown in the image), the density is approximately 1.4667 kg/m³.

How to Calculate Mass and Density from Volume

Density Calculation: The density is calculated using the formula:

\(\rho = \frac{m}{V}\)

Mass Calculation: The mass is calculated using the formula:

\(m = \rho \cdot V\)

Where:

\(\rho\) = Density (kg/m³)
\(m\) = Mass (kg)
\(V\) = Volume (m³)

Example Calculation: Let’s calculate based on the image example with a volume of 15 m³ and a mass of 22 kg:

Density: \(\rho = \frac{22}{15} \approx 1.4667 \, \text{kg/m³}\), which matches the image result.
If density were provided (e.g., 1.4667 kg/m³), mass would be \( m = 1.4667 \cdot 15 \approx 22 \, \text{kg} \).

Use the form above to input your volume and optionally a density. If density is provided, the calculator will compute the mass; otherwise, it will compute the density if mass is provided separately.

Density Units and Conversions

The SI unit for density is kilogram per cubic meter (kg/m³). Other convenient units include:

Gram per cubic centimeter (g/cm³): 1 g/cm³ = 0.001 kg/m³
Kilogram per liter (kg/L): 1 kg/L = 1000 kg/m³
Gram per milliliter (g/mL): 1 g/mL = 1000 kg/m³

Imperial units for density include:

Pound per cubic foot (lb/cu ft)
Pound per cubic yard (lb/cu yd): 1 lb/cu yd ≈ 0.037 lb/cu ft
Ounce per cubic inch (oz/cu in): 1 oz/cu in = 108 lb/cu ft
Pound per gallon (US) (lb/US gal): 1 lb/US gal ≈ 7.48 lb/cu ft

The calculator displays density in multiple units for convenience.

Standard Densities

Density varies depending on the material. Some standard densities at room temperature (20 °C) include:

Water: \(\rho \approx 1000 \, \text{kg/m³}\)
Air: \(\rho \approx 1.225 \, \text{kg/m³}\)
Iron: \(\rho \approx 7870 \, \text{kg/m³}\)
Gold: \(\rho \approx 19300 \, \text{kg/m³}\)

Use this calculator to compare the density of your material against these standards by inputting its volume and mass or density.

What is Volume?

Volume is the amount of space an object occupies, typically measured in cubic meters (m³), cubic centimeters (cm³), liters (L), cubic feet (ft³), or cubic inches (in³). It is a key parameter in calculating density and can be measured or calculated based on the object’s dimensions.

FAQs

How do you calculate density from volume and mass?

To calculate density:

Measure the mass \( m \) in kilograms (kg).
Measure the volume \( V \) in cubic meters (m³).
Use the formula: \(\rho = \frac{m}{V}\).

How do you calculate mass from volume and density?

To calculate mass:

Measure the volume \( V \) in cubic meters (m³).
Measure the density \(\rho\) in kg/m³.
Use the formula: \( m = \rho \cdot V \).

What is the density for a volume of 15 m³ and mass of 22 kg?

The density is \(\rho = \frac{22}{15} \approx 1.4667 \, \text{kg/m³}\), as shown in the image.