What is Number Density

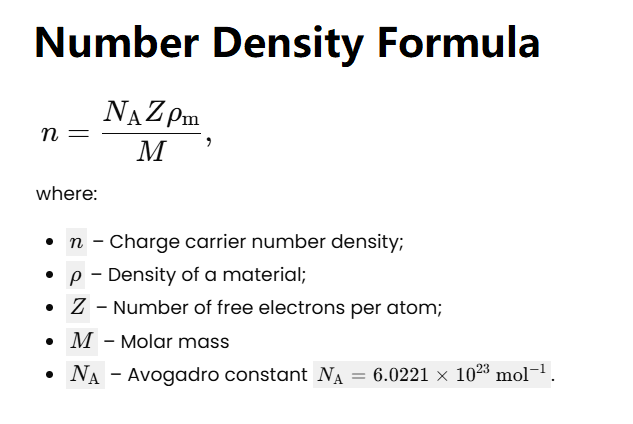
Number density refers to the number of particles (in this case, free electrons) per unit volume in a material, typically measured in electrons per cubic meter (m³) or cubic centimeter (cm³). For metals, the number density of free electrons is crucial for understanding electrical conductivity. It depends on the material’s density, molar mass, and the number of free electrons per atom, which varies depending on the material’s atomic structure.

This calculator allows you to compute the number density of free electrons by selecting a material or entering custom values for density and molar mass, along with the number of free electrons per atom.

How to Calculate Number Density

Number Density Calculation: The number density of free electrons is calculated using the formula:

\(n = \frac{\rho \cdot N_A \cdot z}{M}\)

Where:

• \(n\) = Number density (electrons/m³)
• \(\rho\) = Density of the material (kg/m³)
• \(N_A = 6.022 \times 10^{23} \, \text{mol}^{-1}\) = Avogadro’s number
• \(z\) = Number of free electrons per atom
• \(M\) = Molar mass (kg/mol)

Example Calculation: Let’s calculate the number density for copper (\(\rho = 8960 \, \text{kg/m³}\), \(M = 63.55 \, \text{g/mol} = 0.06355 \, \text{kg/mol}\)) with 1 free electron per atom:

1. \( n = \frac{8960 \cdot (6.022 \times 10^{23}) \cdot 1}{0.06355} \approx 8.486 \times 10^{28} \, \text{electrons/m³} \).
2. In cm³: \( 8.486 \times 10^{28} / 10^6 = 8.486 \times 10^{22} \, \text{electrons/cm³} \).

Use the form above to select a material or input custom values, along with the number of free electrons, to compute the number density.

Material Properties

The calculator includes the following materials with their respective densities and molar masses:

Material	Density (kg/m³)	Molar Mass (g/mol)
Aluminum	2700	26.98
Copper	8960	63.55
Gold	19300	196.97
Silver	10500	107.87
Magnesium	1740	24.31
Tungsten	19300	183.84
Mercury	13550	200.59
Nickel	8900	58.69
Platinum	21450	195.08
Iron	7870	55.85

Select "Custom" to input your own density and molar mass values.

What is the Number of Free Electrons?

The number of free electrons per atom (\(z\)) depends on the material’s atomic structure. For metals, this is typically the number of valence electrons that contribute to electrical conduction. Common values include:

• Copper, Silver, Gold: 1 free electron per atom
• Aluminum: 3 free electrons per atom
• Magnesium: 2 free electrons per atom

Input the appropriate value for your material to calculate the number density.

FAQs

How do you calculate number density?

To calculate the number density of free electrons:

1. Determine the material’s density \(\rho\) in kg/m³.
2. Determine the molar mass \(M\) in kg/mol.
3. Determine the number of free electrons per atom \(z\).
4. Use the formula: \( n = \frac{\rho \cdot N_A \cdot z}{M} \), where \(N_A = 6.022 \times 10^{23} \, \text{mol}^{-1}\).

What is the number density of copper with 1 free electron per atom?

For copper (\(\rho = 8960 \, \text{kg/m³}\), \(M = 63.55 \, \text{g/mol} = 0.06355 \, \text{kg/mol}\), \(z = 1\)):

\( n = \frac{8960 \cdot (6.022 \times 10^{23}) \cdot 1}{0.06355} \approx 8.486 \times 10^{28} \, \text{electrons/m³} \).

Number Density Calculator© - All Rights Reserved 2025