 Home
Home
 Back
Back
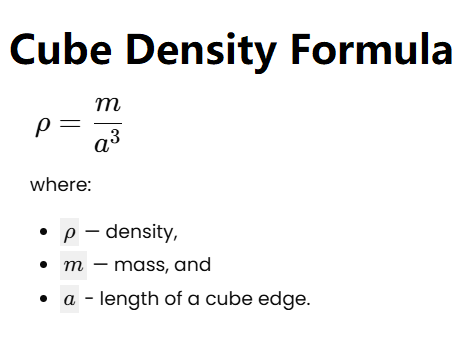
This calculator computes the volume of an object and the side length of a cube given its density and mass. The fundamental relationship is derived from the density formula:
\( \rho = \frac{m}{V} \)
Where:
Rearranging for volume:
\( V = \frac{m}{\rho} \)
For a cube, the volume is related to the side length \( a \) by:
\( V = a^3 \)
Thus, the side length is:
\( a = V^{1/3} \)
Input the density and mass, select your preferred units, and the calculator will compute the volume and cube side length.
Suppose you have a mass \( m = 120 \, \text{kg} \) and density \( \rho = 25 \, \text{kg/m³} \):
\( V = \frac{m}{\rho} = \frac{120}{25} = 4.8 \, \text{m³} \)
Side length of a cube:
\( a = V^{1/3} = 4.8^{1/3} \approx 1.687 \, \text{m} \)
If you prefer the volume in cm³:
\( V = 4.8 \cdot 10^6 = 4800000 \, \text{cm³} \)
Side length in cm:
\( a = 1.687 \cdot 100 \approx 168.7 \, \text{cm} \)
The calculator supports multiple units:
Results are displayed in the selected units for convenience.
How do I calculate the density of a sugar cube?
Measure the side length \( a \) of the sugar cube using a ruler, then weigh it on a scale to get the mass \( m \). Use the formula \( V = a^3 \) to find the volume, and calculate density with \( \rho = \frac{m}{V} \). Alternatively, input the mass and volume into this calculator to get the density directly.
How do I find the density of a wooden cube?
Since wood density varies, measure the cube’s side length to calculate volume (\( V = a^3 \)), then weigh it to find the mass. Use \( \rho = \frac{m}{V} \) to compute density. You can also input mass and volume into this calculator for quick results.
What is the density of a cube with a side of 2 ft and a mass of 5 lbs?
First, calculate the volume: \( V = a^3 = 2^3 = 8 \, \text{ft}^3 \). Then, use \( \rho = \frac{m}{V} = \frac{5}{8} = 0.625 \, \text{lb/ft}^3 \). You can also input the side length and mass into this calculator to verify the density.
How do I use this calculator to find the side length of a cube?
Input the density and mass of the cube, then select your preferred units. The calculator will compute the volume using \( V = \frac{m}{\rho} \), and then find the side length with \( a = V^{1/3} \). The result will be displayed in your chosen length unit.
Can I calculate the volume of a non-cubic object using this calculator?
Yes, this calculator computes volume using \( V = \frac{m}{\rho} \), which works for any object shape as long as you know its density and mass. However, the side length calculation assumes the object is a cube (\( V = a^3 \)).
What if my density or mass is in different units?
The calculator supports multiple units: density (kg/m³, g/cm³, lb/ft³), mass (kg, g, lb), volume (m³, cm³, ft³), and length (m, cm, ft). Select the units of your input values, and the calculator will handle conversions automatically to provide accurate results.